What if you want to build the most energetic particle accelerator in the world and you want it to fit into a pre-existing 27 km circumference tunnel?
Parts list:
- Source of particles (in this case, protons)
- Pre-accelerators
- Shovel (understatement)
- Lots of helium (why?)
- What else?


Let's get at one aspect of the problem:
How do we keep protons at 7 TeV (max design energy) running around in a 27 km circle?
Let's do the simplest possible classical calculation, imagining the LHC is a big cyclotron in a uniform magnetic field with no need to worry about relativity (that is, model it as what it really isn't). Here are some notes:


Try this calculation out and see what you get!
This may seem like a small field...because it is. We need to take into account the relativistic momentum of the protons (max 7 TeV/c = 7000 GeV/c):


Do the calculation: it will still understate the magnetic fields needed by more than an order of magnitude. The reason is that we cannot have a single uniform magnetic field. Rather, the proton paths are bent by over 1000 dipole magnets placed around the ring
Let's bring in somre resources:
Now what if the dipoles each runs with an 8 Tesla magnetic field and we imagine it is basically a powerful electromagnet made from #8 gauge wire? (It isn't, of course.) What current would it run?
More notes:

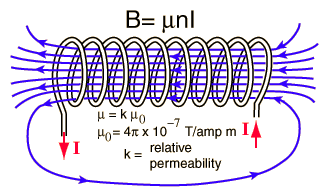
(You can find out how to get the diameter of a particular gauge of wire here.)
This puts us in the ballpark...and it is a lot of current. So why do we need all that helium again?
Here is what we do with all those high-energy, high-momentum protons:
- We collide them for a maximum collision energy of 14 TeV.
- We use large detectors to measure what comes out of the collisions.
- We use conservation laws to work backwards from those measurements to understand their parent particles that were made in the collisions.
- We use triggering to look for the most interesting physics.
- We send the data out for analysis to a worldwide grid.
Speaking of detectors...

